Importance of Pascal's Triangle

Mathematical knowledge presents different dimensions. On one hand, it's a discipline abstract that allows us to understand and describe the world around us. Secondly, it is an auxiliary science that becomes a basic tool for other scientific disciplines and branches of knowledge (economics, medicine, architecture, engineering, etc.). Finally, it is a formal science with innumerable curious aspects.

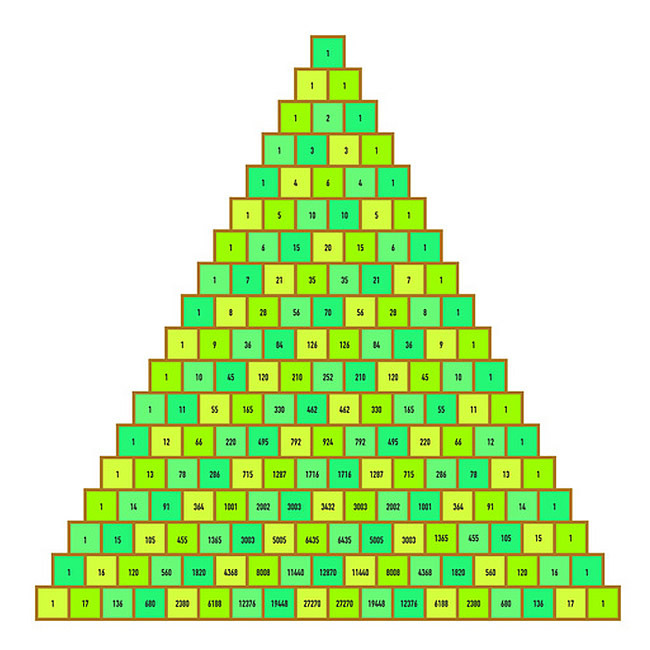
Pascal's Triangle, also known as Tartaglia's Triangle, is one of the most unique mathematical descriptions known.

A simple triangle made with numbers and that has allowed us to obtain all kinds of arithmetic information

The characteristics and properties of Pascal's Triangle were made known for the first time in 1654 with the edition of the book "Treatise on the arithmetic triangle" by the French philosopher and mathematician Blaise Pascal.

In an equilateral triangle (with three equal sides) a number system is distributed. At the top of the triangle appears the first row with the number 1 and all successive rows have the number 1 at both ends.

The next row is formed as follows: 121. From the following an operation is performed math: the sum of 1 + 2 and the sum of 2+1, with which the following sequence is obtained: 1331.

Then the same operation is performed, that is, 1+3, 3+3 and 3+1, with which a new numerical row (14641) is obtained.

The triangle can be increased to infinity following the aforementioned guideline.

What can we find in it?

– Allows you to order the binomial coefficients, that is, the number of objects that can be chosen within a set. Suppose we have four colors: blue, yellow, green, and red. Next we ask how many ways I can choose two of them. The result is as follows: red-green, red-yellow, red-blue, green-yellow, green-blue, and yellow-blue, making a total of six possible combinations of two colors.

The six possibilities are indicated in Pascal's Triangle, since the number 6 is the one found in the middle of the numerical sequence of the fifth row of the triangle (14641).

– If we add the numbers from each of the rows, the different powers of two appear (2, 4, 8, 10…).

– If we take any diagonal as a reference, the triangular numbers appear (for example, 1, 3, 6, 10, 15, 31). A triangular number is one that is equal to the sum of several integers (for example, 15 is equal to the sum of 1+2+3+4+5).

– Mathematicians claim that Pascal's Triangle contains vast numerical information.

– The Newton binomial coincides with the information of this curious triangle, since the coefficients of the Newtonian binomial appear in the succession of numerical rows described by Pascal.

– Finally, the elements of the famous Fibonacci sequence also appear in Pascal's Triangle.

Fotolia Images: Photopic, Archivist