Example of Equivalent Fractions

The equivalent fractions are those that, when compared, have different numerators Y denominators, but they are worth the same. They are characterized because, in each one, numerator and denominator are in a relationship determined.

For example:

These four fractions are equivalent because there is an equal relationship between all of them. The numerator and denominator are in a 1 to 2 relationship.

• In 1/2, this relationship is seen immediately.
• In 2/4, the relationship is the same: from 1 to 2, only the numerator and denominator would be multiplied by (2).
• In 3/6, the relationship is the same: from 1 to 2, only the numerator and denominator would be multiplied by (3).
• In 4/8, the relationship is the same: from 1 to 2, only that the numerator and denominator would be multiplied by (4).

Since we observe it, we can say that the pattern there is is: "In each of the equivalent fractions, the numerator and the denominator are related, which is multiplied or divided by a certain number."

If we multiply or divide the numerator and denominator of any fraction by the same number, the result we obtain is an equivalent fraction.

Examples of equivalent fractions

Next, series of equivalent fractions are written, classified according to how they are obtained, into two categories:

• Equivalent fractions by multiplication
• Equivalent fractions by division

Equivalent fractions by multiplication

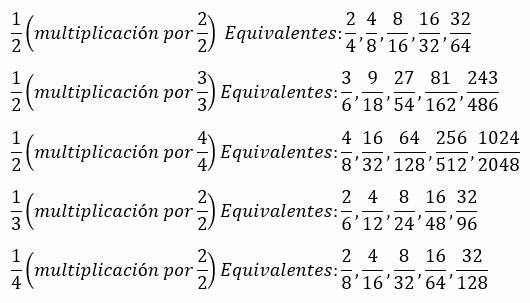
Equivalent fractions by division

How to check that two fractions are equivalent?

To verify that two fractions are equivalent, we must multiply cross: numerator of the first by denominator of the second, and denominator by opposite numerator. The product must be the same. If there are different results, the fractions are not equivalent.

For example:

Now you know how to correctly identify equivalent fractions.

To learn all about fractions, visit:

• Fractions
• Proper fractions
• Improper fractions
• Mixed fractions
• Conversion of fractions