Example of the Law of Signs

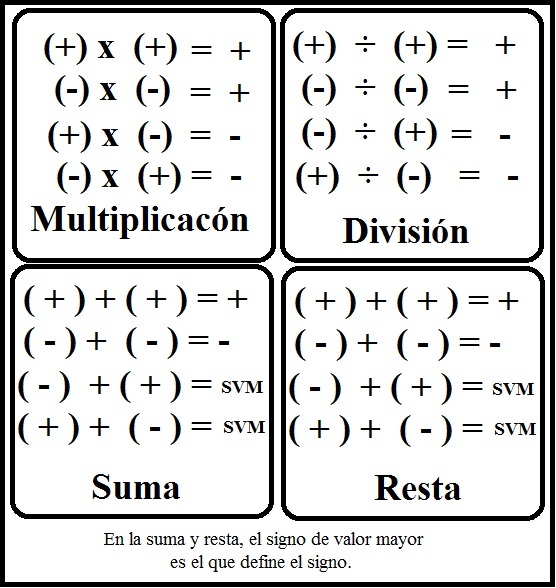
The Law of Signs is the law that establishes how the signs of the numbers behave at the time of mathematical operations. If this law is applied correctly, a correct result is guaranteed in any addition, subtraction, multiplication and division that is done. This law is concerned with the meaning that the numbers would have on a number line, and uses the signs "+" and "-", the sign "+" being named as "plus" and corresponding to positive numbers; and the sign "-", named "minus", corresponding to negative numbers.

Indications can be established for the Law of Signs, which will be as follows for Additions and Subtractions:

"In equal signs, there will be accumulation"

"In opposite signs, the values ​​are counteracted"

Law of signs in addition

In the case of the Add operation, if the two numbers are positive, they will accumulate, and it can be said that the result will have a larger, positive value.

(+18) + (+20) = +38

And, if there is a sum where a number is negative, the values ​​will counteract like this:

(+18) + (-20) = -2

In this case, the (-20) caused us to stay negative. We load more on the negative side because 20 is a value that exceeds 18.

When both signs are negative, the result is a higher negative number; there is also accumulation:

(-6) + (-14) = -20

Law of signs in subtraction

In the operation of the Subtract, the sign "-" affects the term that follows, changing it to the opposite. The operation is carried out at the end, adding the values ​​in a sum:

(+15) – (+6) = (+15) + (-6) = +9

(-15) – (+6) = (-15) + (-6) = -21

(+2) – (+18) = (+2) + (-18) = -16

(-10) – (+6) = (-10) + (-6) = -4

To know what sign the result will have in a Subtraction, it is important to pay attention to the two key steps:

Step 1: Change of sign of the term that follows the sign.

Step 2: Check which sign has the highest number. This way we will know if we are inclined towards a result with a positive or negative value.

Indications can be established for the Law of Signs, which will be as follows for Multiplication and Division:

"If there are positive equal signs, the result will have the same sign"

"If there are negative equal signs, herethe result will also be Positive "

(+3) x (+6) = +18

(-2) x (-4) = +8

(+36) ÷ (+6) = +6

(-150) ÷ (-10) = +15

"If the signs negative a number appears odd of times, the result will have a sign negative”

(-8) x (-4) x (-10) = -320

(-420) ÷ (-10) ÷ (-7) = -6

"If the signs negative a number appears couple times, the result will have a sign positive” 

(-100) x (-3) = +300

(-99) ÷ (-11) = +9

10 Examples of addition with the law of signs:

In addition, the numbers are added preserving the sign they have. If they have the same sign, the values ​​accumulate. If the signs are opposite, the values ​​are offset towards the highest value number:

(+8) + (+20) = +28

(+10) + (-2) = +8

(-24) + (+5) = -19

(-18) + (+14) = -4

(+7) + (-13) = -6

(+9) + (-21) = -12

(-5) + (-25) = -30

(-14) + (-28) = -42

(+10) + (-5) = +5

(+10) + (-9) = +1

Examples of Subtraction with Law of signs:

In Subtraction, the sign of the number that follows the sign of the operation is changed, and the numbers are added:

(+8) - (+20) = (+8) - 20 = -12

(+10) - (-2) = (+10) + 2 = +12

(-24) - (+5) = (-24) - 5 = -29

(-18) - (+14) = (-18) - 14 = -32

(+7) - (-13) = (+7) + 13 = +20

(+9) - (-21) = (+9) + 21 = +30

(-5) - (-25) = (-5) + 25 = +20

(-14) - (-28) = (-14) + 28 = +14

Examples of Multiplication with Law of signs:

In Multiplication, if both signs are equal, the sign will be Positive in the result:

(+8) x (+2) = +16

(-10) x (-2) = +20

(-2) x (-5) = +10

(+18) x (+2) = +36

And if the signs are opposite, the result will be negative:

(+7) x (-3) = -21

(+9) x (-2) = -18

(-8) x (+2) = -16

(-4) x (+8) = -32

Examples of Division with Law of signs:

In Division, as in Multiplication, if both signs are equal, the result will have a positive sign.

(+8) ÷ (+2) = +4

(-10) ÷ (-2) = +5

(-9) ÷ (-3) = +3

(+12) ÷ (+2) = +6

And if the signs are opposite, the result will be negative:

(+7) ÷ (-1) = -7

(+10) ÷ (-2) = -5

(-20) ÷ (+2) = -10

(-16) ÷ (+8) = -2