Pascal's Triangle Example

It is known as "Pascal's triangle" or "Tartalian triangle" to a numerical formation that was better known and disseminated by the mathematician of French origin Blaise Pascal and for which it bears the name of Pascal's triangle.

Several mathematical applications are given to this training, among which the extraction of the Newton's binomial, which allows us to obtain the prime numbers.

Finally, we highlight in the following list three mathematical processes obtained in Pascal's triangle:

1. Sum
2. Stick de Hoquey (sum of diagonals with result at the end)
3. Prime numbers
4. Fibonacci sequence

Conformation.- The construction of Pascal's triangle is descending, starting at the top and descending infinitely.

Examples of Pascal's triangle:

Horizontal sum.- The horizontal addition is performed by adding the numbers from left to right or from right to left, thus obtaining the result.

Hockey Stick.- This consists in that the sum of the numbers that begins at a higher end, culminates with the final number that is the result as seen in figure 2.

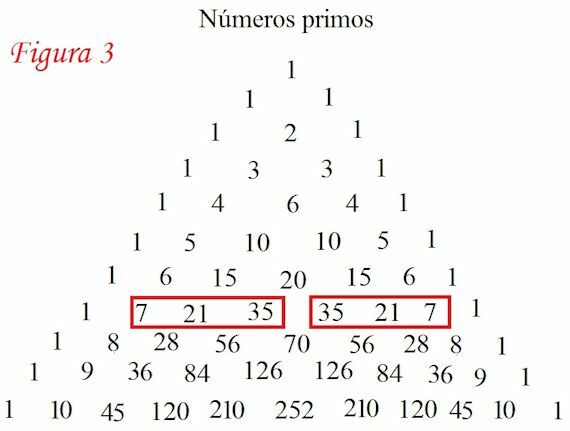
Prime numbers.- These occur when the first number in a row is prime, the following numbers will be divisible by the number with the exception of the number 1 as can be seen in the figure 3.

Fibonacci sequence.- The Fibonacci sequence is called the result obtained by adding two numbers in order to obtain the next number, so Let's add:

Do not forget to leave your comments.