Definition of Electrical Resistance

Evelyn Maitee Marin
Industrial Engineer, MSc in Physics, and EdD

The electrical resistance, or also called a resistor, is an electronic component whose function within the circuits is to oppose the passage of electrical current through it. For many authors, resistance refers specifically to the physical property, which is expressed in ohms (Ω), and the word resistor is used when it comes to the component.

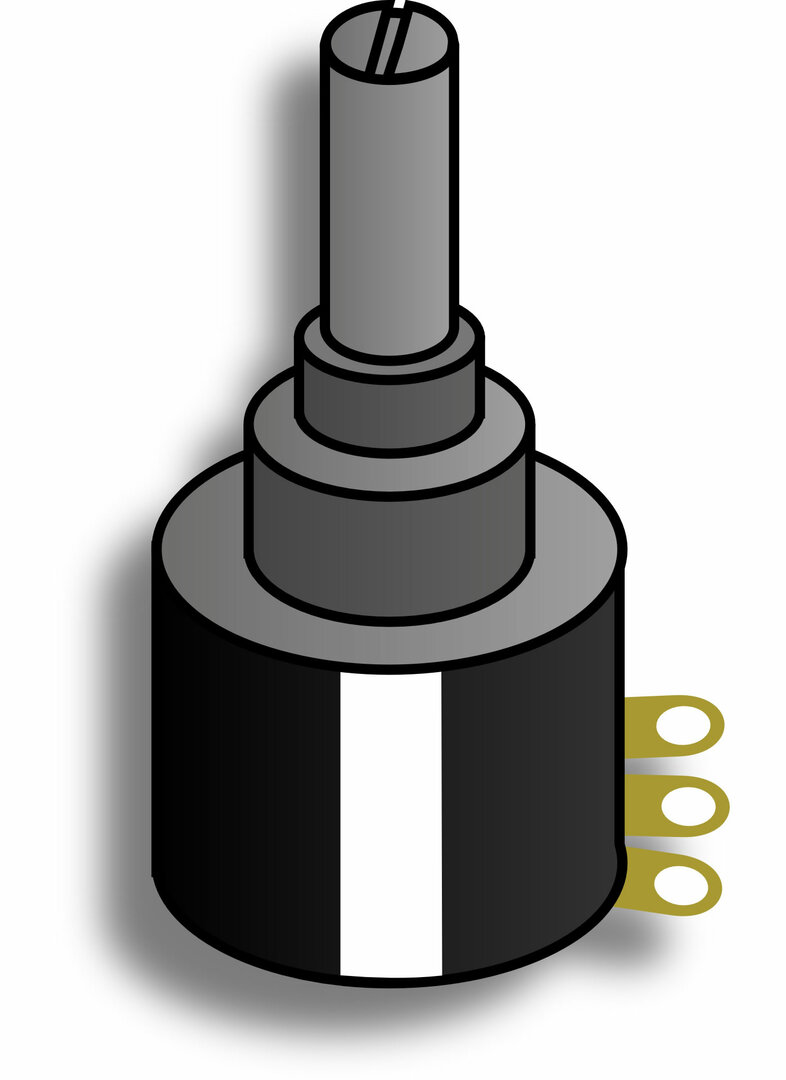
Potentiometers or rheostats are variable resistors that allow obtaining between a terminal extreme and intermediate, a fraction of the resistance between the two extreme terminals of the component.

The property of resistance is the opposite of conductivity, and various factors determine the resistance of a material, the main ones being the nature of the material (metals, ceramics, etc), its geometry and the temperature at which it is find. The electrical resistance of a conductor can be calculated using the expression:

\(R = \frac{{\rho \cdot L}}{s}\)
Where,
R: Electrical resistance (Ω)
ρ: electrical resistivity
S: cross-sectional area of ​​the conductor
L: conductor length

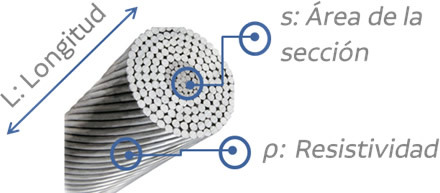
The resistance of a conductor depends on its resistivity, length, and the area of ​​its cross section.

resistance association

In the resolution of electrical circuits that involve resistances, it is generally necessary to determine the the equivalent resistance of an association of resistors, whose most common combinations are in series and parallel.

series resistors: These are two or more resistors that are connected by a single common terminal. By this type of associations, when they are connected to a voltage source, the same intensity of current (i) circulates.

The equivalent resistance for a series connection is determined by adding each of the resistances in the array:

\({R_{equi – series}} = \mathop \sum \limits_{i = 1}^n {R_i}\)

For example, if you have three resistors in series, as shown, the equivalent resistance will be:

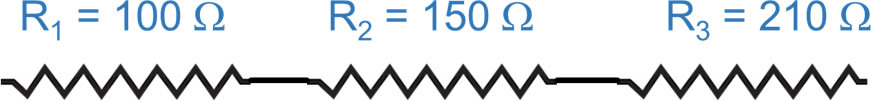
\({R_{equi – series}} = 100 + 150 + 210\)

R._equi-series = 460 Ω

resistors in parallel: the arrangements in this case are identified because two or more resistors have their two terminals in common. When these types of connections are present in a circuit powered by a source, the voltage (V) that occurs across the terminals of all resistors is the same.

The inverse of the equivalent resistance of a parallel arrangement is obtained by adding the inverses of the resistances.

\(\frac{1}{{{R_{equi – parallel}}}} = \mathop \sum \limits_{i = 1}^n \left( {\frac{1}{{{R_n}}}} \right)\)

For example, if you have three resistors in parallel as shown in the image, the equivalent resistance will be:

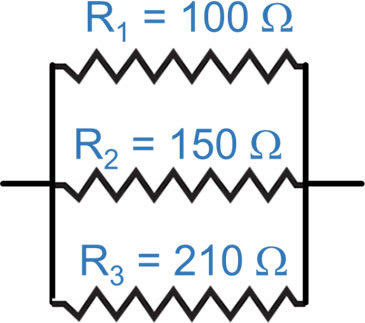
\(\frac{1}{{{R_{equi – parallel}}}} = \frac{1}{{100\;}} + \frac{1}{{150\;}} + \frac{1 }{{210\;}}\)

\({R_{equi – parallel}} = 46.67\;\)

Note: if only two resistances are available in parallel, the equivalent resistance is obtained by the quotient of the product of the two resistances divided by their sum.

Knowing the value of the resistor and its voltage or current, the missing parameter can be determined from Ohm's law:

V = i. R.

Color code

All materials have a certain electrical resistance, and in electronics, this component comes in different presentations, such as ceramic resistors, which use a color code to indicate their nominal value and tolerance, or variable resistors or potentiometers. The following table shows the different values ​​according to the resistor color code:

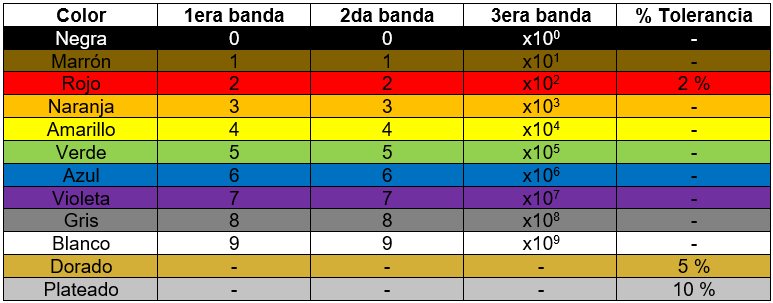
The resistance contains four colored bands: the first two figures express the resistance coefficient, the The third color is the multiplying factor in base 10 power, and the fourth band represents the percentage of tolerance.

Considering the sequence of colors shown in the image resistor, it can be determined that its value is (15×10² ± 5%) Ω

resistance applications

Virtually all electrical and electronic circuits use electrical resistors to obtain the variations of voltage or current intensity according to the requirements of the circuit.

Electronics has evolved and every day the components become more compact and integrated in order to save space and expand their features.

The most common fixed electrical resistors are carbon or film, wound or wire, and fusible alloy.

When a current passes through an element with resistance, a power is generated in it, which is usually dissipated as heat, so it is usually used this principle in many residential and industrial applications where it is required to produce heat, such as electric stoves or ovens industrial.