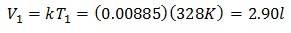Cube Root Example

The Cube root It is the inverse operation of cubing a number, (which is the multiplication of a number by itself three times). In other words, the cube root is used to find the number that multiplied by itself three times, gives as a result the number from which we are taking the root.

When we multiply a number by itself three times, we say that we cube that number.

For example, when cubing the number 4, we do the following:

4³ = 4 X 4 X 4 = 64

The cube root is used to find the number that raised to the cube gives us as a result the number from which we are extracting the root. We can understand this operation as the operation with which, knowing the volume of a cube, we can calculate how much one of its sides measures.

The cube root symbol is formed with the radical symbol and the root indicator, which is the number 3:

³√

The cube root of the numbers less than 1000, is included in the numbers that include the units:

1³ = 1

2³ = 8

3³ = 27

4³ = 64

5³ = 125

6³ = 216

7³ = 343

8³ = 512

9³ = 729

10³ = 1000

For numbers greater than 1000, we must take into consideration that the cube of a two-digit number, that is, with tens and units, will produce numbers in thousands. This characteristic is important to take into account, since to calculate the cube root of large or decimal numbers, the periods in which the number is divided will be three digits.

Another important detail that we must take into account in order to calculate the cube root is that to calculate each period (that is, each division in thousands) the The number to be cubed can be expressed as the sum of the two figures, that is, as a binomial of the form d + u, where the letter d is the tens, and the u the units. We can understand this by developing the polynomial, and in parallel substituting the values:

(d + u)³ = d³ + 3d²u + 3du² + d³

12³ = 10³ + (3)10²(2) + (3) (10)2² + 2³ = 1000 + 600 + 120 + 8 = 1728

123 = 12 x 12 x 12 = 1728.

To finish these previous ideas, it remains to explain that when calculating the cube root, we will not use the term d³, since it is the first term that we calculate, and as each period goes down, we will only use the 3d terms²u, 3du² and u³, from which we will add their values ​​and subtract them from each term. When solving, the result of 3d²u will multiply it by 100, that of 3du² we will multiply it by 10 and the result of u³, we will leave it at that. This is the step-by-step explanation of how to calculate the cube root:

To extract the cube root of a number

How to get the cube root of a number?

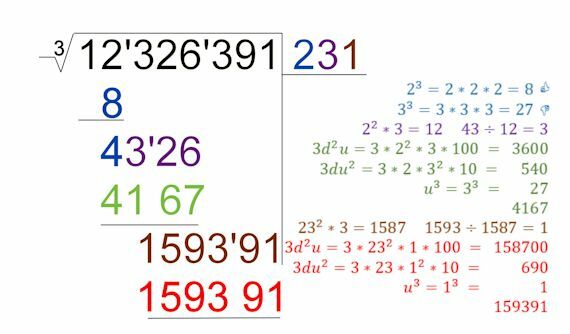
FIRST STEP. (Black color) We start by dividing the number into periods. Each period will be made up of three numbers. In the whole numbers they will be counted from the decimal point, to the left in the whole numbers, and to the right in the decimal numbers. We will calculate the cube root of 12326391. We divide the number into periods and place it inside the radical symbol.

SECOND STEP. (blue color) We calculate the cube root of the first period (which is the one that is furthest to the left), looking for the number that cubed is equal to or closer to the number we are looking for, without going over and we subtract.

THIRD STEP. (purple color) We lower the next period and place it next to the result of the subtraction. We separate the last two numbers from the right. we square the number we have as a root, and we multiply it by three. We divide the number that was left separated in the result by the number that we just obtained, and the integer result of the division is the next number in the root.

FOURTH STEP. (green color) From the number that we have as a root, we separate the units (which will be the u value of our equation) and the remaining numbers will be the tens. Next, we determine the values ​​of 3d²u, 3du² and u³, we add them and subtract the result.

FIFTH STEP. (Brown color). We lower the next period together with the result of the subtraction and separate the last two figures. We square the root and multiply by three. We divide the number that was left by the result of the multiplication that we just did and the whole result is the next number in the root.

STEP SIX. (Red color). We again separate the units and the tens. If the root has three or more digits, when separating the units, the value of d (the tens) can contain two or more digits. We determine the values ​​of 3d²u, 3du² and u³, we add their results and subtract.

Steps five and six are repeated until the result is zero if the root is exact or the remainder is reached if it is inaccurate. The same procedure is followed when the number to which the root is taken has decimal numbers.

Examples of cube roots:

³√ 232608375 = 615

³√ 614125 = 85

³√ 74088 = 42

³√ 82312,875 = 43,5

³√ 1953125 = 125

³√ 160103007 = 8543

³√ 485587,656 = 78,6

³√ 946966,168 = 98,2

³√ 860085351 = 951

³√ 9993948264 = 2154

³√ 183250432 = 568

³√ 274625 = 65

³√ 363994344 = 714

³√ 15625000 = 250

³√ 627222016 = 856

³√ 1838,26563 = 12,25

³√ 2863288 = 142

³√ 418508992 = 748

³√ 465484375 = 775

³√ 6028568 = 182

³√ 14348907 = 243

³√ 1367631 = 111

³√ 35937 = 33

³√ 2263,5713 = 13,13

³√ 3944,312 = 15,8

³√ 1728000 = 120

³√ 0,421875 = 0,75

³√ 1906624 = 124

³√ 33076161 = 321

³√ 314709522 = 680,2